 |
 |
TSP Showcase Project (SCTP1907) at the University of Sydney
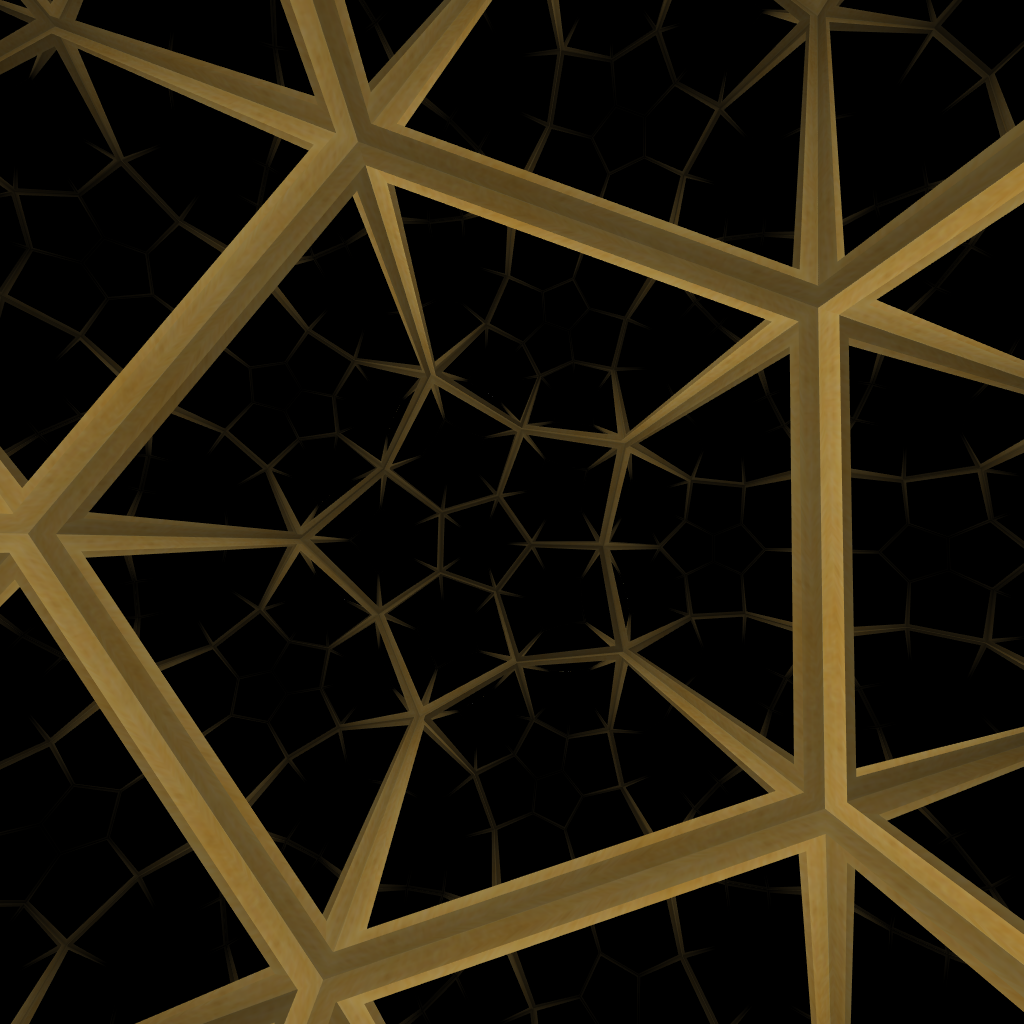
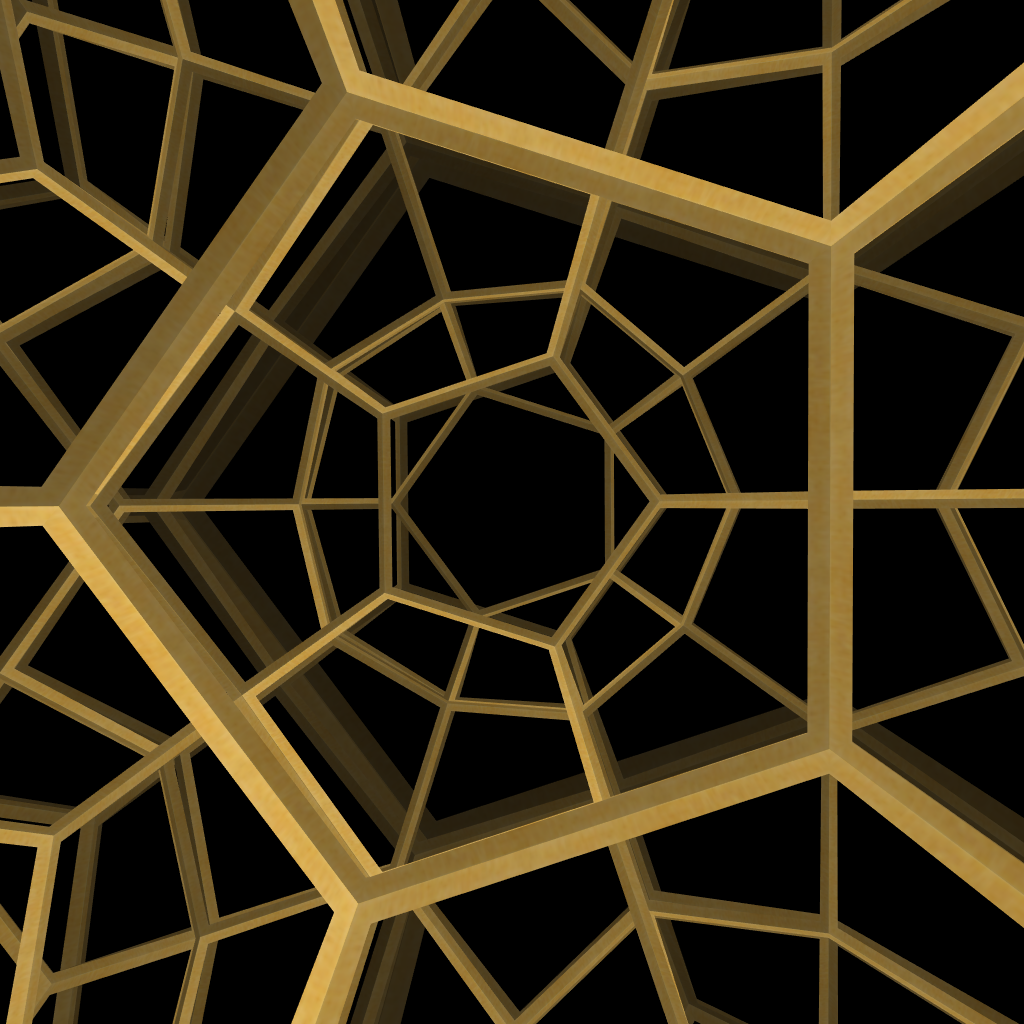
Shapshots of the hyperbolic and spherical dodecahedral tesselations from Jeff Weeks' Curved Spaces
What is a symmetry of an object? Children learn to cut a heart out of paper by folding a sheet of paper in half and then cutting out half of the pattern. The resulting shape has a reflectional symmetry - the reflection is a transformation that preserves the shape of the heart. In more general terms, symmetry of an object is a transformation that preserves some (usually implicit) structure. A standard brick wall has a repeating pattern that can extend over the whole plane, and the same is true for the patterns on most wallpapers. The paper heart and the letter "A" have the same type of symmetry: only one reflection preserves the shape. It is a theorem that there are just 17 types of repeating patters on the plane!
The implicit structure on the plane is called euclidean. Instead of the euclidean plane, one can consider symmetrical patterns on the sphere. It turns out that there are 14 types of such patterns, and it is fun to try and draw different patterns on table tennis balls. There is another class of patterns that fall into the same category, but less obviously so. They are drawn on a disc, but the objects, even though they repeat, appear to change in shape and size. An example of this is the woodcut "Angels and Devils" by M.C. Escher. The angels close to the centre appear much larger than the angles near the boundary of the disc. They are in fact of the same shape and size if one gives the disc the right implicit structure: if one views it as the so-called hyperbolic plane. The geometry on the hyperbolic plane is so rich that it allows infinitely many types of repeating patterns!
Usually, crystallography is concerned with the 3-dimensional analogue of the euclidean plane. You can imagine space made up of neatly stacked cubes, and in total there are in fact 230 types of tesselations of euclidean space. This project will be concerned with spherical and hyperbolic analogues of this, and software as well as pipe cleaners will assist you in visualising these 3-dimensional spaces and to develop an intuition for their geometric properties.
Both 3-dimensional spherical space and 3-dimensional hyperbolic space can be tesselated with regular dodecahedra (just as euclidean space can be tesselated with cubes). This project will explore the symmetry and structure of these two tesselations with a view towards shedding some light on two open research questions concerning the tesselation of hyperbolic 3-space.
Weekly meetings start in Week 5 (time and place TBA), and the project concludes in Week 12.
The following books have been put on reserve in the library, and I have indicated which Chapters may be relevant to this project. I do not expect you to work through the whole chapters or sections, but rather to look at them with your project in mind.
These geometry games by Jeffrey Weeks can help to gain some intuition about spaces of dimensions 2 and 3:
You are especially encouraged to explore the Curved Spaces software and the hyperbolic games, and to go through the study questions, which are accessible through the games' help menu.
The heading indicates a main theme for the week. As stated above, I do not expect you to work through the whole chapters or sections, but rather to look at the concepts, results and techniques with your project in mind. Some key notions will be introduced in Week 5 in a friendly manner, so that you can use your imagination to formulate some aims and ideas of what you'd like to be able to "see" by the end of the project. These aims will be expanded and refined throughout the project, as you learn more mathematics around the theme as well as the techniques that you need to realise your aims.